Ball Mill Scale-up by this method is known as collision energy based population balance model. The appropriateness of this model is shown for 38-cm ball mill. Comminution in ball mills account for a significant amount of power consumption in mineral processing plants. Therefore, accurate modeling of the process is extremely important to optimize the power consumption, which can lead to substantial economic benefit. In the past few decades, a plethora of research work has been published that dealt with ball mill scale-up. The first systematic scale-up procedure was proposed by Bond. By means of a set of grinding experiments conducted in a laboratory mill a parameter known as “Bond’s work index” is determined. This parameter is an indicator of the material strength subject to the mill operating conditions. Material work index is then scaled up for larger size mill and correlated with mill power, mill dimensions and operating conditions. In the later stage all the scale-up methodologies were formulated on the basis of the population balance model, also known as selection/breakage function model. All these methods suggest a way to determine the selection and breakage function suitable for modeling the product of industrial ball mills from the tests conducted in laboratory scale mills.

Population balance models predicts the product size distribution of tumbling mills by the following differential equation-

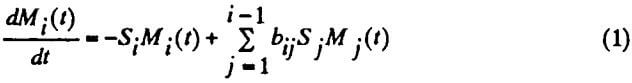
Where, Mi(t), is the mass of particle in the i-th size class at the time instant tiSi, stands for the selection function or breakage rate of size class i and bij indicates the traction of material of class j appearing in class i due to breakage. In earlier research works it is established that breakage function does not depend upon the grinding environment and also it can be normalized with respect to size. Therefore, the task of scale-up is to find out a method to determine the selection function for larger mills.

The scale-up procedure using the “specific energy hypothesis”. The hypothesis suggests that selection function can be normalized with respect to the mass-specific power input to the mill. The obtained specific selection function becomes just a material constant. Function is expressed as follows:

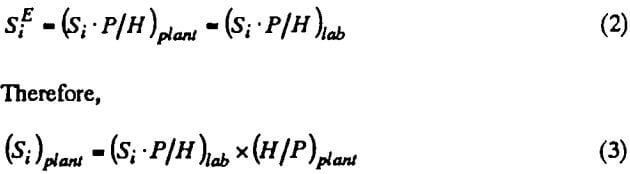
Here, SiE is the specific selection function, P, is the mill power draft and H is the mass hold-up of the mill. In a laboratory, Si, is determined by grinding the same type of material in a small mill under identical operating condition that are desired for the large mill.

Then selection function for plant mill is calculated with the help of equation 3.

Later on Austin (1984-b) included the effect of ball size distribution, mill speed, particle filling and slurry density individually in his model by means of empirical correlation. Both the methods assume that the collisions also scale-up in the same way like mill diameter and other operating conditions from lab mill to plant mill. This very assumption raises lots of questions. Because for same fraction of mill speed an industrial mill rotates in a slower speed and balls travel a much longer distance than a lab mill. These factors will certainly alter the interaction among the individual balls. The fundamental difference in charge motion between a small and large mill will introduce some discrepancies in the results of the traditional scale-up methods.

The discussion so far certainly indicates a need for a scale-up procedure based upon the collisions oocurring within the mill. The net power supplied to the mill is expended in the collisions of various types (ball/ball and ball/mill-shell). There is a spectrum of collisions taking place at various energy levels and these collisions are also responsible for breakage of the mill charge. The lower energy collisions produce a coarser progeny size distribution, while the higher energy collisions are the reason behind the finer product size. The intensity of collisions will also affect the amount of mass broken per unit time or the selection function. Since, different milling conditions generate dissimilar patterns in collision intensities, a scale-up procedure based on the information regarding collisions possesses more credibility. Therefore, researchers are putting more effort on the fundamental study of particle breakage and modeling ball mill grinding based on collisions.

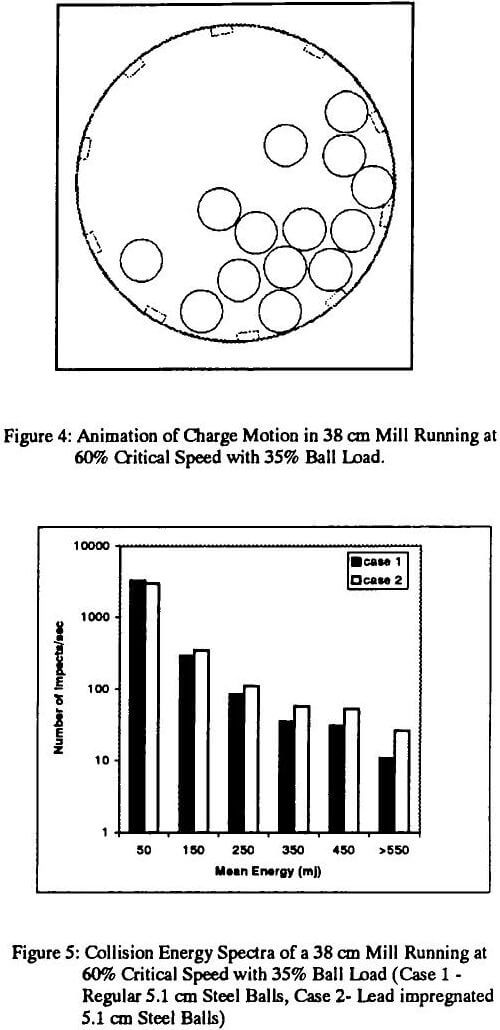
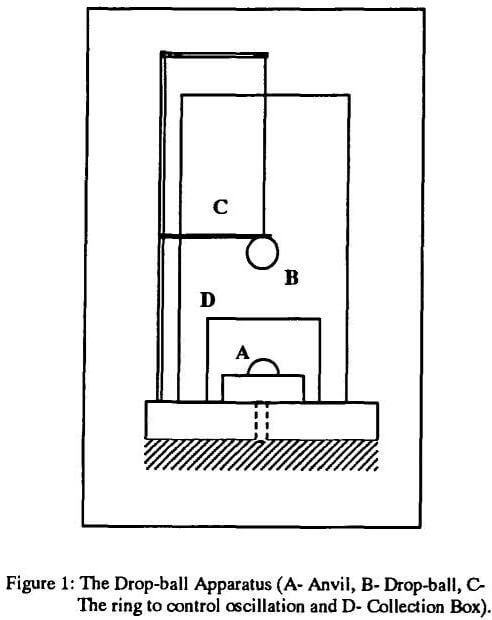
In this work a new procedure for estimation of mill product size has been proposed. The essence of this method is the determination of the collision energy spectrum valid for a particular set of milling conditions by means of numerical simulation. A grinding model, in the line of classical population balance model, is developed. The relevant parameters for the model are experimentally determined using a drop-ball apparatus. The model is then validated against data obtained from lab scale ball mills.