Table of Contents
The technical excellence of separation achieved in a mineral concentration process, or any other process where two constituents of any kind are physically separated from each other, is expressed uniquely and quantitatively by the Separation Efficiency:
Es = (R – Rg)
where R is the percentage of the valuable constituent and Rg the percentage of the waste appearing in the concentrate. The Separation Efficiency meets all the usual requirements of a true efficiency and is independent of the nature of the process used. It can be visualized as the percentage of the feed material that, in effect, actually undergoes complete separation while the rest of the feed is distributed randomly and unchanged into the two separation products.
Mineral Concentration Processes and their Evaluation
The overall concentration process may be subdivided into one of liberation, which severs the bonds between unlike mineral species, and another of separation, which sorts the resulting liberated particles into two products, a concentrate, rich in mineral “A”, and a tailing, lean in mineral “A”.
A perfect mineral concentration process is represented by the idealized scheme shown in Figure 1 in which liberation and separation are not differentiated. The feed mixture consists of two types of minerals which become completely liberated early in the process and then sorted by type. All the mineral particles of type “A”, which respond uniquely to the selection forces acting in the ideal separator, report to the concentrate while all the mineral particles of type “non-A” report to the tailing. The result is two perfect products, pure “A” and pure “non-A”.
Some basic requirements to be fulfilled by an efficiency expression designed to assess concentration operations have been set forth by Stevens and Collins and by Douglas. From these and other considerations, it is concluded that a suitable separation efficiency should:
- Be a function of feed and product amounts and compositions;
- Be applicable to any physical separation of one material from another and be adaptable to multi-component systems;
- Be zero for mere sampling operations and 100% for a perfect separation
- Be independent of the means used to accomplish the separation;
- Be independent of economic considerations;
- Be the same for the separation of the first constituent from the second as for the separation of the second from the first when a two-component feed is treated in one operation;
- Be physically significant in a way that can be visualized.
To sum up, two basic efficiency expressions applicable to the separation of two-component systems are dominant in the literature on the subject of evaluation of concentration processes. These are the “Separation Efficiency”, Es, and the “Concentration Efficiency”, Ec, which are related by:
Ec = Es (Actual enrichment ratio)/(Maximum enrichment ratio) = Es(c/cm)
The other proposed methods of evaluation are either not true efficiencies or are relative values of Es or Ec that are based on standard rather than theoretically perfect separations.
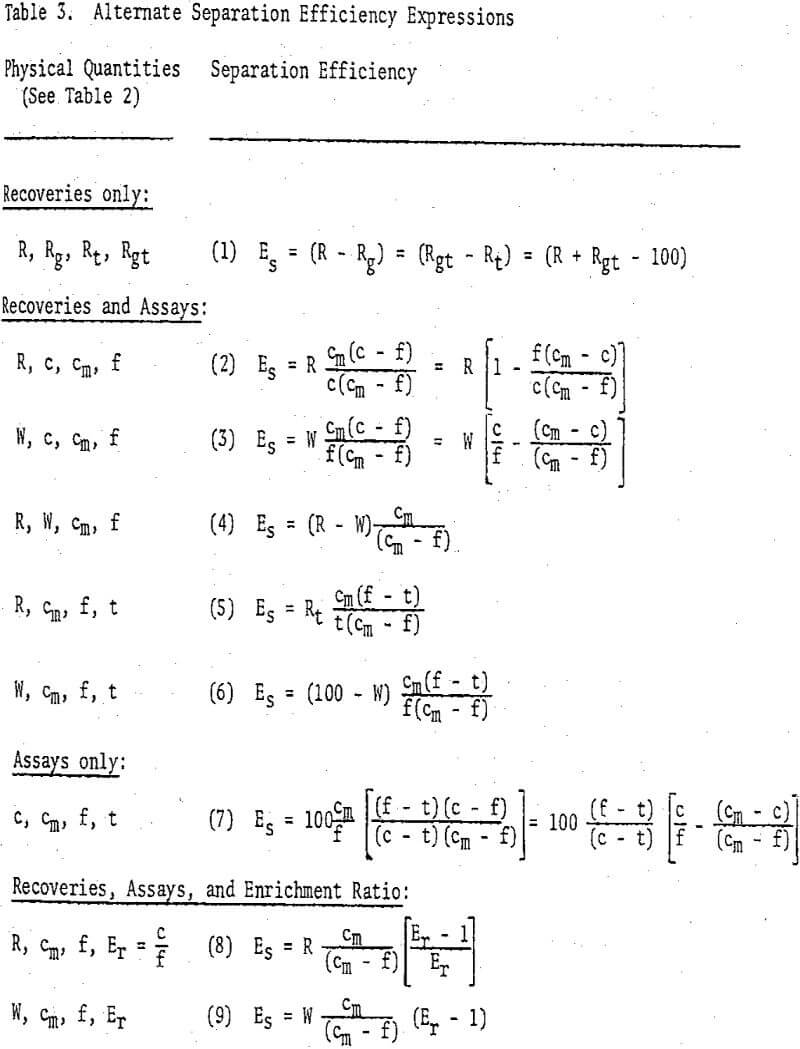
The various forms which Separation Efficiency expressions can take are listed in Table 3 for convenience. Similar expressions for Concentration Efficiency are easily derived from these as noted above.
The relative merits of Separation Efficiency and Concentration Efficiency may be determined by comparing them on the basis of the seven basic requirements listed earlier.
Applications of Separation Efficiency
A finely ground artificial magnetite ore was subjected to a series of Davis magnetic tube tests, and the resulting concentrate grade and recovery data were plotted to yield the Magnetic Separation Characteristic (MSC) curve. Several iso-efficiency curves for this magnetite ore are drawn in to show their relationship to the MSC curve. For cm = 72.36% (Fe in Fe3O4) and f = 38% (Fe in feed),
R = 0.475 (Es) (c/c – 38)
The calculated average grade of particles being rejected and the cumulative grade of tailing are also plotted against total iron recovery. It is interesting to note that the ordinate through the point where the reject grade crosses the initial feed grade-line intersects the MSC curve at its point of nearest approach to the iso-efficiency curve for Es = 80%, that is, where Es for this particular operation is maximum.
In a recent study of the separation and simultaneous recovery of iron and manganese from a chemically reduced manganiferous iron ore, selection of optimum conditions frequently required close appraisal of similar magnetic separation test results. The application of Separation Efficiency,